A Quantum Continuation for Moore’s Law?
While engineers grapple with the end of Moore’s law, rudimentary prototypes of a completely new type of machine — the quantum computer — have been making news. These devices exploit the properties of quantum mechanics — in particular, phenomena known as superpositions and entanglement — to speed up certain calculations. Whereas the standard ‘classical’ computer deals with bits, each a definite 0 or 1, a quantum computer uses qubits, whose interlinked quantum states combine 0-ness and 1-ness in varying proportions and phases.
Interest in quantum computing exploded precisely 25 years ago, when mathematician Peter Shor, now at MIT, devised a quantum algorithm for factoring numbers that would be exponentially faster than any known conventional algorithm. But could anyone build a sizable quantum computer to perform such feats in practice? The technical challenges were, and are, immense. Qubits must be exquisitely isolated from stray disturbances; and conversely, they must interact with one another with great fidelity.
Skeptics, most notably the late Rolf Landauer of IBM, argued in the 1990s that fundamental problems such as errors introduced by disturbances and less-than-perfect technology posed insurmountable obstacles to realizing the vision. His skepticism early on helped to strengthen the field. For instance, with qubits, established techniques for fixing an occasional flipped bit would be worse than useless; they would destroy the very quantum states they were meant to protect. In response, researchers devised elaborate schemes for quantum error correction. Meanwhile, laboratories painstakingly developed an eclectic variety of crude qubits, such as individual atomic ions.
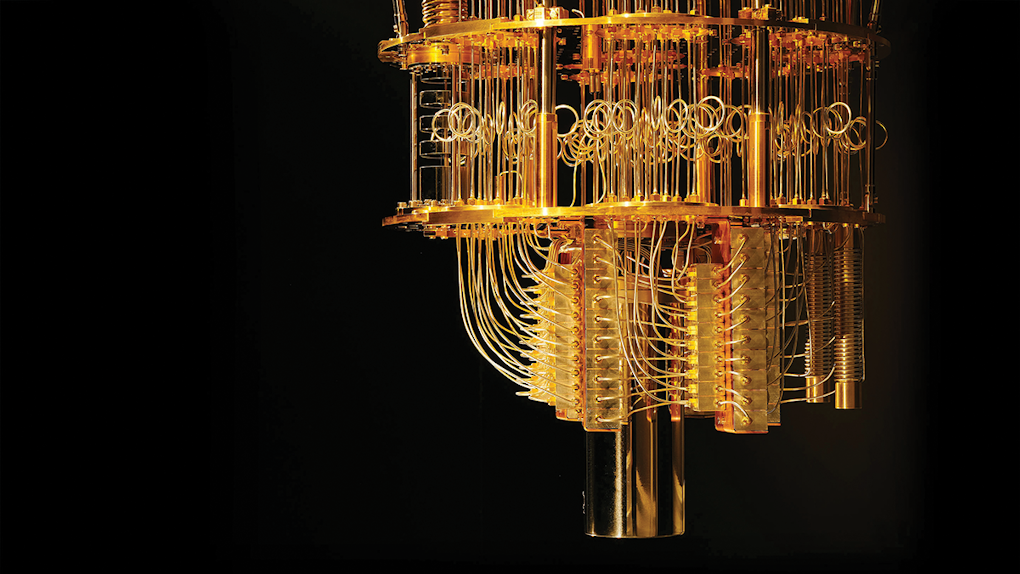
Today, those long years of preliminary research are being translated into engineering designs that connect small but growing numbers of qubits into primitive working systems. The field is leaping ahead, with IBM, Google and Intel announcing devices with dozens of qubits made of superconducting circuits. Quantum supremacy, the demonstration of a calculation that a conventional computer could not match, may be just around the corner.
To be clear, quantum computers are not the answer to the end of Moore’s law. Their theoretical ability to wipe the floor with ordinary computers is limited to very specific tasks, such as factoring and simulating quantum chemistry or materials. And fully realizing their potential requires qubits with vastly improved fidelity and stability so that error correction can be demonstrated and made useful in larger systems. But while these daunting challenges remain, disproving the skeptics now seems like a matter of time and further hard work rather than an unresolved question for the distant future.
